

Provide the Setting the Stage With Geometry Reference Sheet: Perimeter, Area, Surface Area, and Volume printable for students who are having trouble remembering which formulas go with which polygon. Step 8: Check for Understanding: Go over all correct answers as a class, referring to the Answer Key: Setting the Stage With Geometry printable. Step 7: Distribute the Setting the Stage With Geometry Worksheet: Pack it Up! What Will Fit? printable and have students complete the worksheet independently.
A square pyramid with a base side length of 12.5 inches and a height of 9 inches. Volume of the square pyramid is 468.75 cubic inches (1/3 x 12.5 x 12.5 x 9). A cylinder with a radius of 9 centimeters and height of 7.25 centimeters (use 3.14 for π and round to the nearest hundredth). Volume of the cylinder is 1,843.97 cubic centimeters (3.14 x 92 x 7.25). A rectangular prism with length 6.5 meters, width 7 meters, and height 12.5 meters. Volume of the rectangular prism is 568.75 cubic meters (6.5 x 7 x 12.5). Step 6: In groups or in pairs, with use of calculators as an option, ask students to calculate the volume of: Demonstrate that the volume of this pyramid is 48 cubic feet (1/3 Show students the volume formula for square pyramids on the poster: V= 1/3 BA h. Be sure to point out the difference between height and slant length, as students might confuse the two. Step 5: Finally, draw a square pyramid on the board with a base length of 6 feet and a base width of 6 feet. Demonstrate how the volume of this cylinder is 113.04 cubic feet (3.14 x 32 x 4 = 113.04). Show students the volume formula for cylinders on the poster: V= π Step 4: On the board, draw a cylinder with a radius of 3 feet and a height of 4 feet. Since the dimensions of the rectangular prism are 3 x 4 x 5, the volume equals 60 cubic units. If necessary, show students the volume formula for rectangular prisms on the poster: V (volume) = l Step 3: Ask students to find a relationship between the lengths of the sides and the volume. If helpful, point out how the unit of measure for area is square units (unit times unit equals unit squared) and for volume is cubic units (unit times unit times unit equals unit cubed). Stress the need for precision when indicating units of measure. Explain how a cubic unit is the unit of measure for volume. Step 2: Ask how many cubes it took to build the prism (60). Note: If you have enough time and an adequate supply of manipulatives, have students construct rectangular prisms, either individually or in groups. Using unifix cubes or a similar manipulative, construct a rectangular prism with height = 3 units, length = 4 units, and width = 5 units. Step 1: Explain to your students that now that they've mastered measuring the surface area of 3-D shapes, they can move on to measuring volume, which is the amount of space inside a 3-D shape. Introduction to Formulas for Finding Volume Optional: Make class sets of the Setting the Stage With Geometry Take-Home Activity: The Perfect Fit printable and the Turn Up the Volume! Bonus Worksheet printable for students to complete as part of the Lesson Extensions. 
Hang a copy of the Setting the Stage With Geometry Classroom Poster printable in your classroom or project it using a computer and projector.Print a copy of the Answer Key: Setting the Stage With Geometry printable for your use.
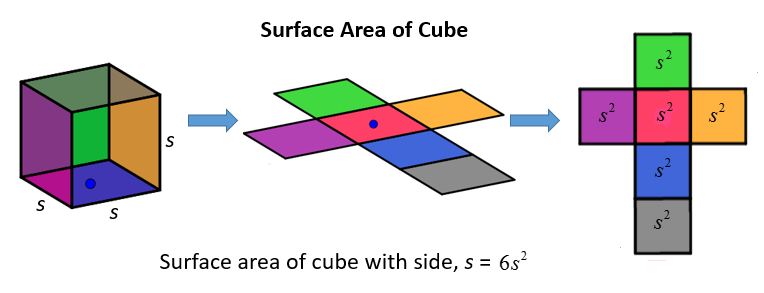
Make class sets of the Setting the Stage With Geometry Worksheet: Pack it Up! What Will Fit? printable and the Setting the Stage With Geometry Reference Sheet: Perimeter, Area, Surface Area, and Volume printable.Optional: Turn Up the Volume! Bonus Worksheet printable.Optional: Setting the Stage With Geometry Take-Home Activity: The Perfect Fit printable.Setting the Stage With Geometry Classroom Poster printable.Answer Key: Setting the Stage With Geometry printable.Setting the Stage With Geometry Reference Sheet: Perimeter, Area, Surface Area, and Volume printable.Setting the Stage With Geometry Worksheet: Pack it Up! What Will Fit? printable.Be able to use formulas to measure the volume of a rectangular prism, a cylinder, and a square pyramid.







 0 kommentar(er)
0 kommentar(er)
